Click image for free 18Mb pdf of this "out-of-print" book

This article and others are included in the 18 chapters of "Rethinking the Atom" a 164 page paperback compilation of articles by JMW $19.95 US list at Amazon Click here  for Amazon.com

Click image for free 22Mb pdf of the book

INTRODUCTION

Back a century ago, scientists worked diligently to explain the spectral data of excited atoms. These spectra included S(harp), P(rincipal), D(iffuse), and F(undamental) lines that were to be addressed. The main thrust of the physical model that continues to this day got its start with Bohr’s orb. From this, “electron house” plans were drawn: a “1-room bungalow” up gradable to a “4-room split level”. Additional stories with 5 or 7-rooms were added to make “electron condos”. The room plans got labeled: s (1), s+p (4), d (5), and f (7) or spdf for short[1].

Pauli mused that there was a problem of which occupant was which when the rooms were double occupied and everybody needed an SS#. Heisenberg, who made significant contributions to what became “quantum mechanics”[2], came to his rescue when he wrote to Pauli in 1926 that e-spin might be the solution to the ortho/para helium phenomenon[3] and thus to Pauli’s exclusion problem. So, “parity electron twins” that occupied the same room (cell, orbital) were created. Some would say that ortho/para helium proved the correctness of the quantum mechanics approach. QM certainly stifled alternative views.

The “quantum mechanical – spin-paired” model still is the accepted convention: n (floor level), ℓ (room shape), m (wing), and s (left or right-handed twin in the double bed) are the notations that define an electrons place in the double-occupancy spdf rooms. While the mirroring of electrons (“mirrored twins”) can be handled by mathematics, it is still not clear just how it happens in our physical world where electrons repel one another. It would have made more sense to have mirrored the rooms that were singularly occupied, but that option was eliminated when the lowest room in any condo had to be a centered sphere.

Scientists are now developing techniques to investigate electrons at the nanometer level[4].  Helium will likely be the next atom studied. To provide an alternate particle approach to the current QM electron orbital model, a “mirrored room” model is applied to para and ortho helium.

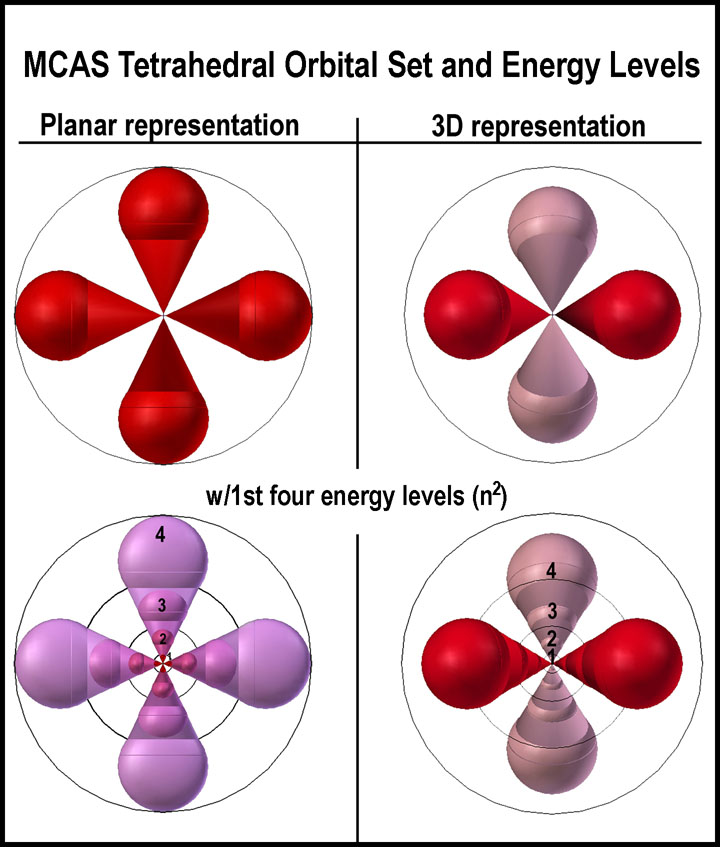
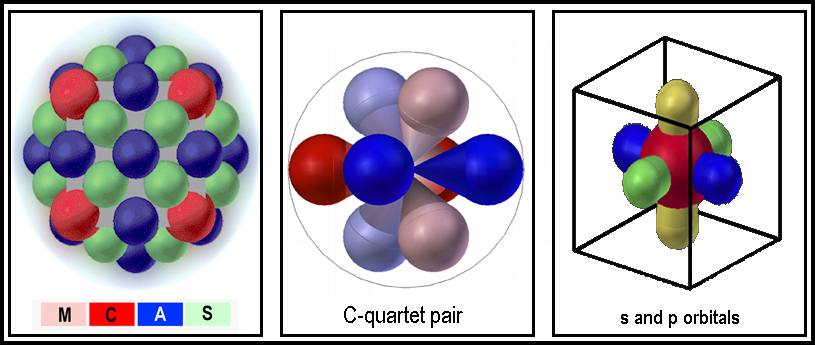
A “MIRRORED ROOM” ELECTRON ORBITAL MODEL

 A “mirrored-room” equivalent of a sphere is easily envisioned. It is simply two, diametrically opposed tetrahedral-lobed spaces with a common center. Each orbital lobe can contain a single electron. When the orbitals are so small that e-repulsions between electrons would make filling all of them prohibitive, only one electron occupies each quartet; this is the case for hydrogen and helium. As the orbital sizes increase (increased energy), all of the orbitals can contain an electron; e.g., neon. Not only does this “mirrored- room” equivalent replace a sphere, it is also equivalent to the s+p, split-level group.  This is the MCAS model[5].

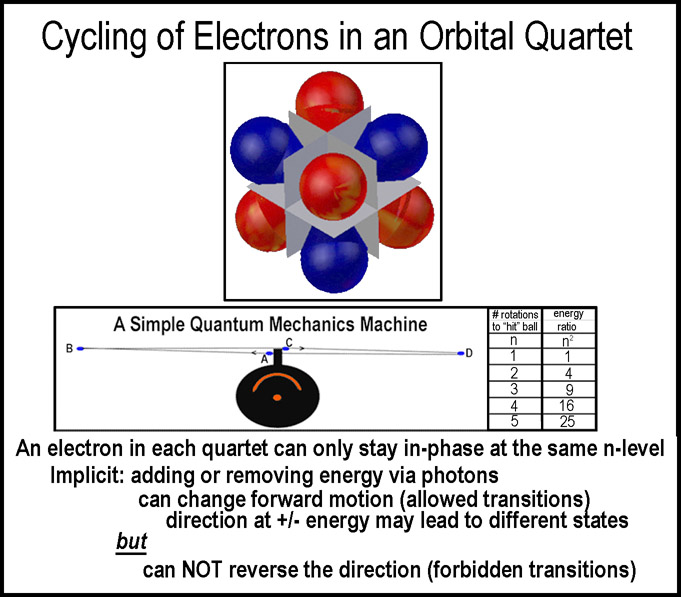A simple quantum mechanics machine (shown at the right and discussed elsewhere[6]) indicates, a priori, why the excitation levels scale by n². Implicit in the model is movement and coordination with the nucleus. The "mirrored-room" orbitals are simply that: orbitals; a different, but simpler set that explains the physical data and the periodic table. They would also be appropriate for non-classical approaches without electron-pairing should stanch adherents to the non-classical get past their objection to the fact that these orbitals can satisfy classical physics as well!

At this point it is important to note the effect of adding and removing energy. Adding energy to or removing it from an electron via photon addition or release is like accelerating and decelerating a car with the gas pedal – there is no effect on direction, per se. A reversal of direction by this action will not happen. Moving to a higher or lower lobe of similar shape is the norm. Moving to another orbital that has another shape is possible, if the direction of the electron at the moment of energy change would send it there. Thus, an electron can NOT move from a RED M-orbital system to a BLUE one or vice versa. This is a “forbidden” transition by a photon change. 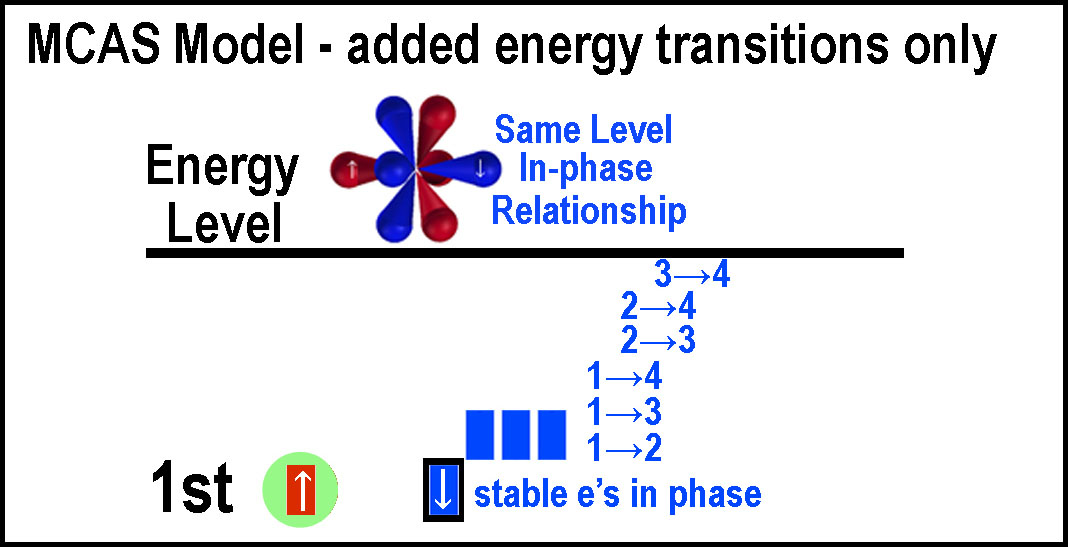To be “in-phase”, two electrons must remain diametrically opposite one another or flowing in the same orbital group. To do this they need to be at the same energy level (n). Otherwise the time to transverse orbital lobes will be different. The “in-phase” state is the lowest for every energy level. Note that there are 3 non-diametric options even at the “same” energy level for the simple opposed quartets – these provide some fine structure before the unstable system moves to the more stable diametrically opposed position through energy loss. They provide a staging point for transitions and may even make one electron appear more energetic than the other.

THE PHYSICAL SPACES OF ELECTRON TRANSITIONS  

The Bohr circles that indicate simple one-electron n-state transitions do not indicate the magnitude of the energy involved in those transitions very well to students by my reckoning. The figure at the right is intended to provide a better feel. Note the tiny little first level circle.

It is all a matter of the transversing time needed to arrive back at the required appointment moment with the nuclear regulatory checkpoint and pass on to the next lobe. The nucleus keeps its roadies in line with firm discipline based on the constancy of the spectral data. This raises the question as to when an electron can add or dump energy: can it occur only when it passes the nucleus, at some other place, at many places, everywhere? Do atoms have drive-through pump and dump stations? Is there “in-the-air” service like the military does for non-landing planes? Knowing how electrons and nuclei interact would be quite valuable information for a more complete understanding.

THE ENERGY STATES OF PARA AND ORTHO HELIUM

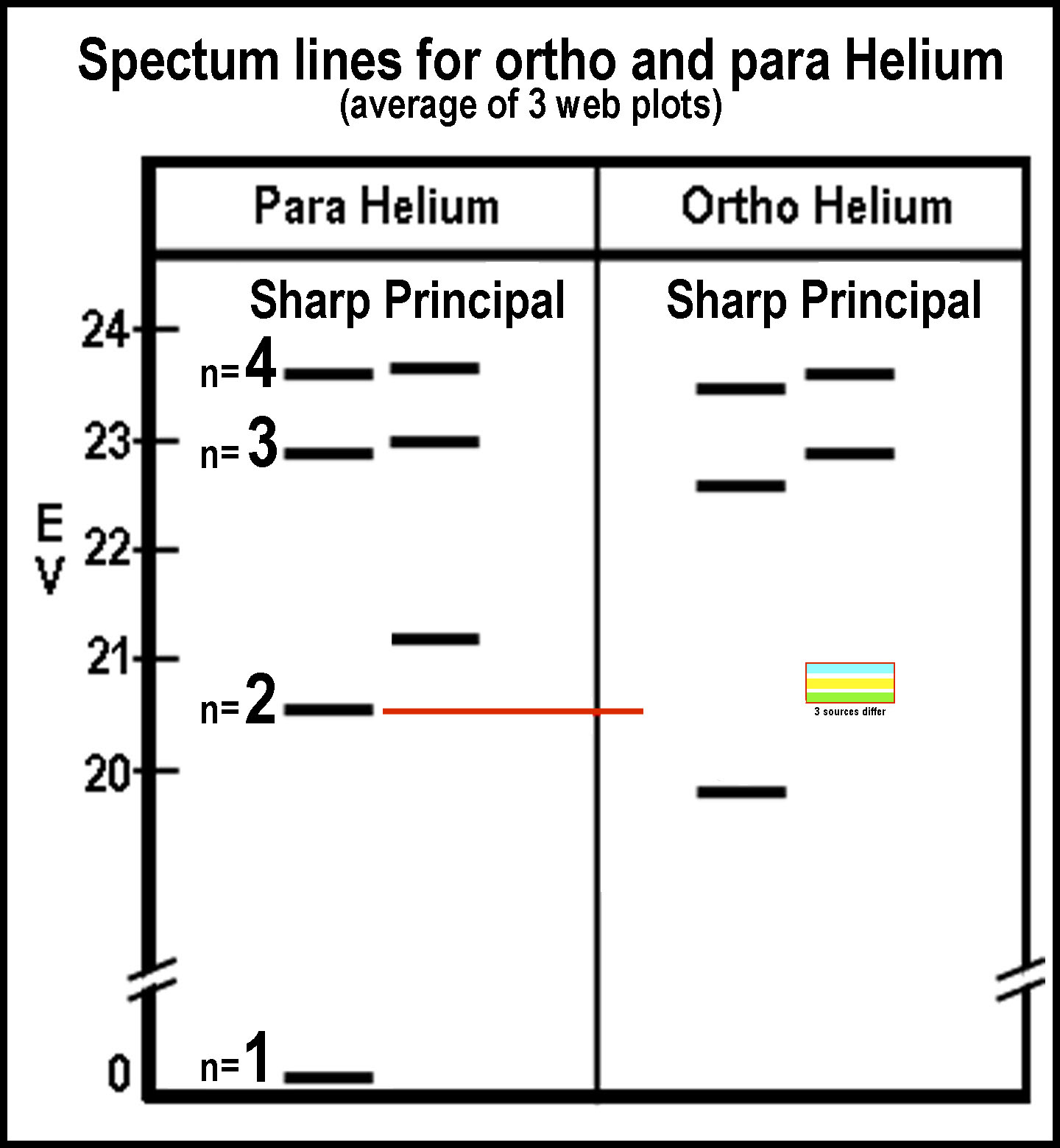The figure at the right is a composite of energy levels from 3 web sources[7]. The data was consistent up to the 4^th n-level, except for the 2^nd level ortho “P”, where none agreed, but differed not greatly. S and P are spectral assignments and, of course, in the quantum mechanical model, they also represent that model’s orbital descriptions. Other energy levels and orbitals are omitted here in order to focus attention on the first levels of energy changes. S and P are handled by the C-orbitals of the MCAS model. The full MCAS model of orbitals is given below: an M-orbital is a mono-occupied C-orbital quartet. The sp orbitals of the spdf model are given for comparison.

Elevation of the ground-state of para-helium to the next lowest energy level occurs in two steps without changes in electron spin. In the first step, one electron is elevated. This leads to a high energy, unbalanced, out-of-phase state. Part of the energy in this state is utilized in the elevation of the second electron. This gives rise to a balanced, in-phase state that has a lower total energy. In the vernacular of chemical reaction mechanisms, the out-of-phase state is a high-energy transition state, or an approximately equivalent meta-stable state. In essence, the energy to promote the two electrons in the n=1 in-phase state to the n=2 in-phase state is less than that required to promote one electron from the n=1 state to the n=2 state. To return to the ground state, the process is reversed.

With ortho-helium, the two electrons are in the same orbital quartet. Readers who are familiar with chemistry will recognize the ortho (adjacent) and para (opposite) placement of the arrows in the two “n=2 in-phase” images in the figure. Having two electrons in the same orbital quartet at the n=1 level is energetically prohibitive. Thus, the lowest state for two electrons in the same orbital quartet is at the n=2 level. This is the reason that lithium starts the second row of the periodic table[8]. As in the case with para-helium, a higher energy state can occur with one of the electrons dropping down to the n=1 level. Unlike in the para case, however, the second electron can not drop down.

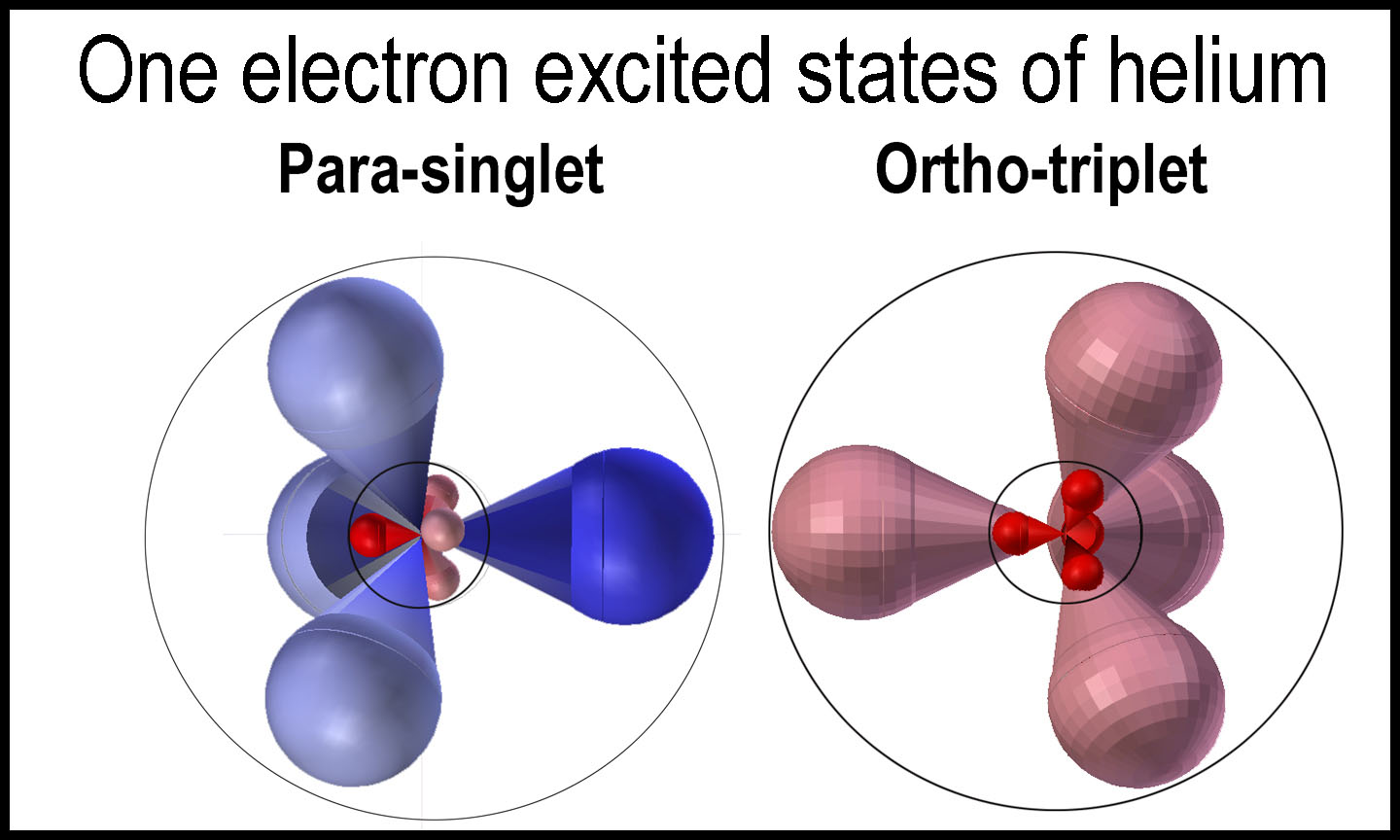At first glance, it would seem strange that the ortho-state is lower in energy than the comparable para-state. Consider the figure on the right. The overall electron interactions are just greater in the para-state than in the ortho state as they move through the orbitals and go through nuclear “supervision”.

The second electron in ortho-helium can not reverse direction under photon action and, thus, allow it to convert to the para arrangement. The conversion of one state to the other will not occur with the simple addition or removal of photon energy as a reversal of direction is needed. Strong magnetic fields are needed to align the electron orbitals (electron flow) to pair them and collision impacts are needed to reverse them.

SUMMARY

The "mirrored-room", MCAS, orbital model provides an excellent explanation for the difference between ortho and para helium. Spin-pairing is accomplished through movement through opposing orbital units rather than through the "mirroring of electrons" in the same orbital space as is done in the spdf model. The MCAS model clearly demonstrates why ortho and para helium are not converted from one to another with photon energy. The model also demonstrates that electrons are operationally connected to one another as they pass by and coordinate with the nucleus. They do not exist as clouds of negativity, although they emit negative fields. Energy levels are related to "coordinated junctions" near the nucleus and scale according to classical acceleration and deceleration laws of electron velocity and their related electrostatic attractions (and repulsions?) with the nucleus and repulsions with other electrons[9]. The MCAS model demonstrates that the lowest (n=1) energy level of atoms can only have two electrons moving in opposite directions (paired) and can not have electrons flowing in the same orbital quartet. This explains why the periodic table begins a new period after helium with a higher energy, more spatially expanded, set of orbitals which does allow additional electrons to occupy the same quartet. The rationale for G.N. Lewis’ "rule of eight" is quite clear with the eight-equivalent lobes of the "mirrored-room" model. The MCAS "mirrored-room" electron orbital model offers the simplest explanation for this and many other atom-atom interactions and does so without having to "hybridize" orbitals to make compounds and "reverse" the actual spin of electrons to effect pairing.

[9] Some might say that electrons "talk" to one another[4]. Since electrons emit electromagnetic fields, they certainly "influence" each other. The nucleus is doing the major controlling, however. The electron "drones" are just responding to its "warping" and the total electrostatic and magnetic fields generated by all of the atomic components. How the nucleus implements its warping influence would be very useful for understanding how these tiny atomic machines operate.